Building Block Units (BBU)

Building Block Units are a family of modules which make it possible to build a huge variety of designs, from typical abstract geometric solids to realistic models of real-world buildings. This versatility is in part possible thanks to the fact that there are a huge number of variants which can all be used together. Apart from about 100 variants I have listed here, countless more are possible, and I often employ one-off units which are only needed for a single design.

Pictures of a few select unit variants:

Examples of models made from BBU units:

Information on other sites

In The Fold, I published Introducing Building Block Units, an article about how I developed this family of designs. It also contains diagrams for a few basic tiles (you can find those also in the instructions below).

Folding Building Block Units

Folding and connecting the units

Due to the large number of different tiles, instructions are split into separate sections for different tile families.

I recommend that you start with folding the simplest tiles and then read on how to connect them:

• BBU family A: plain tiles
• Connecting the units and different connection methods

Then, move on to try out more complex variants:

• BBU family B: stripes
• BBU family C: diagonals
• BBU family D: squares and other polygons
• BBU family E: stars and crosses
• BBU family F: arrows
• BBU family G: bumps and protrusions
• BBU family P: windows and doors
• BBU family Q: roofs
• BBU family R: balconies
• BBU family Z: miscellaneous

Folding with crease patterns

Instructions for most tiles of BBU family are in the form of crease patterns (CPs). This medium is often used for complex origami models made from a single sheet of paper, but I have not seen them used for modular origami much so far. Crease patterns take some time getting used to, but they make it possible to explain advanced folds in little space and after a while they become quite convenient to read. You can find lots of useful information about crease patterns on the web, in particular on the pages below:

• Robert J. Lang’s page on crease patterns
• Crease patterns at origami resource center
• How to fold Box Pleated CPs by Gerwin Sturm

Crease patterns which you can find below were made by folding the units and then tracing the relevant folds with a pen. Helper lines can often be seen as untraced creases. They may be helpful in finding out how to construct particular folds, but for most beautiful effect, consult the section “Avoiding unnecessary creases” below. Some diagrams contain extra tips for constructing non-obvious creases.

Unique tile numbers and tile families

There are lots of different Building Block Units. Most models consist of an inner core which gives them bulk and external tiles which are used to decorate the model. In order to introduce some order to the dozens of different tiles, I assigned each tile an unambiguous identification code (there are too many to use descriptive names conveniently). Each code consists of a letter which is the tile’s family (e.g. family B is “stripes”), and a running number within the family. The division of tiles into families is there in order to make finding tiles needed for a particular kind of model easier. However, the division is only rough and based on the external look of the tiles. As a result, tiles whose folding sequence differs by just a single crease may end up in different families.

Variants and modifications

Since there are so many different tiles, some of them differ only a little. Actually, they form a continuum where each tile can be modified slightly and then some and it is hard to put a sharp boundary to where variations of one tile type end and a new design begins. In general, I consider tiles which have “the same set of creases” but possibly moved relative to each other, to be variants of the same tile. For example in tile E1 (cross), the cross can be made broader or narrower by making creases at different points along the side of the paper. Some modules are chiral (not the same as their mirror image) and I consider both the left and right version to be variants of the same unit. When a crease or two are added to a model without changing its appearance much, I consider this a modification. An example would be folding a unit along its diagonal for assembly such as in the spiked icosahedron model or bending A4 connector modules as needed for a particular model. I usually indicate variants in model descriptions and modifications only if they are not obvious. Some tiles which I consider different and have assigned separate codes could be considered modifications of a single design (e.g. Q14 and Q15) but I included separate diagrams for them in order to highlight the construction methods for their particular proportions. Again, there are no sharp boundaries.

Pretty much any flat tesselation can be transformed into a BBU tile by folding down the edges to make flaps. In most cases this would require paper larger than the one used for inner cubes.

Two-colored units

Most units only display one side of the paper, but a few, for example D2, D4, D17, D18 and E10, display a color-change effect when folded from duo paper.

Arbitrary crease positions

A number of units allow for making creases at an arbitrary position. In the crease patterns such arbitrary coordinates are marked with the letters x, y, z and so on. The same arbitrary distance is marked with the same letter.

Arbitrary folds often determine the relative sizes of flaps. For most external tiles, it is good to make flaps of roughly equal size. In the case of internal tiles (usually A1), it may be beneficial to make flaps with significantly different sizes. This makes less likely situations where two neighboring flaps just barely overlap. Such flaps may cause the paper to curl and be hard to flatten out. It is much better when flaps that meet on one face are either both significantly smaller than half the unit length (no overlap at all) or both significantly larger (big overlap).

In some models, the arbitrary crease is a design decision which determines e.g. how wide a stripe is going to be.

Attaching external tiles to the inner core

Many models have an internal core made of cubes folded from A1 units which is then covered in external tiles which make the model more stable and improve its look. These external tiles are attached in a matter similar to the one used when folding business card cubes: flaps of the external tile are inserted below the flaps of modules building the inner core.

Most models have two flaps which leaves little choice. Others have four flaps which allows either folding two of them below and using only the other two for attachment or putting an extra A2 tile below which makes then attaching with all four flaps possible. Some units have small flaps and all four are needed to hold the unit well in place. Since the direction of flaps in the internal cubes alternates between even and odd rows by 90 degrees, external tiles with two flaps tend to be rotated by 90° every other row. In some tiles (e.g. D6), there are only two flaps, but they can be made either in one direction or in the perpendicular direction so it is possible to have all external tiles pointing one way without the need of putting additional A2 tiles for rotation below.

There are tiles which can not be easily rotated and creating a tile which looks the same on the outside but has flaps located in the perpendicular direction requires a completely different crease pattern than the original. Many stripe patterns in the B family have this property.

Some tiles can’t be attached directly to the inner cubes and require another tile to be used as a base (for example the swan roof Q19 requires tile Q18 as a base).

Squares and rectangles

Building Block Units are by default made for folding cubes and have square faces, but with minor modifications they can be used to make rectangular faces and rectangular cuboids. Not all tiles can be transformed this way but many of the plain and striped patterns (A and B families) can.

For a rectangular cuboid with dimensions a × b × c, units with face sizes a × b, a × c and b × c (two of each) have to be prepared. This is easiest accomplished by making the first unit with selected sizes and then using that unit as a template to copy the desired length onto the other units. It is easiest to construct rectangular units corresponding to the square unit A2. An example is shown below.

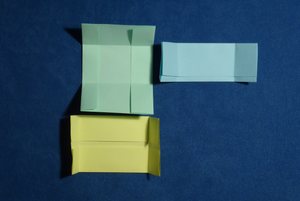
Avoiding unnecessary creases

For many models, there is an obvious way of folding which is quick but which leaves some helper creases visible. In almost all cases, it is possible to avoid these unnecessary creases in order to end up with a clean fold. Crease patterns help a lot since they only show significant creases. For a clean unit, try to fold only those lines which are shown in the crease pattern. Helper lines can often be hidden: instead of folding a line through the whole unit, make just a tiny pinch near the paper’s edge. Edges often become the flaps which are hidden once the unit is attached to the model. I plan to expand this section some time in the future.

For this tutorial, crease patterns were folded without much regard for avoiding helper creases. In contrast, complete tiles were folded as to avoid unnecessary creases.