Sturdy Edge Module (StEM)

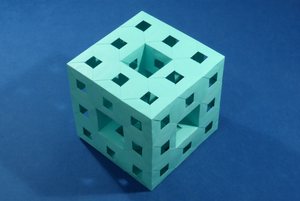
StEM is a very versatile module which can be used to make a number of very different objects. Three units can build a triangular 45-degree pyramid, so anything that can be made with Sonobe units can also be made with StEM. In addition, objects with square, triangular and hexagonal faces can be made. The latter two require a small adaptation of the units and result in faces which are not flat: the triangles/hexagons will stick outwards or point inwards a bit. Since the hole in the middle of the square face is ⅓ of the edge length, this unit is well-suited for making Menger Sponges. In most uses, StEM is an edge unit, but when folded in half along the shorter axis, it can be used as a face unit which behaves quite a bit like Sonobe unit (alternatively, you can see it still being an edge unit but with the edge going along the shorter instead of the longer axis of the module).

This unit is quite simple and after inventing it, I found out that a number of other people had had the same idea before. See the model’s page for details.

Instructions for other peoples’ versions (in print media)

I got the information below courtesy of Dirk Eisner:

Tomoko Fuse’s […]. She has another folding, which is equal to your SEU, in Unit Origami Wonderland. This book is in Japanese. She named it something like 45°-60°-unit. Here the second end of the module is different. Francis Ow named it 90-degrees unit. He has a diagram in his booklet Modular Origami. He started with a 1/6 segmentation, but then it is similar to your unit. With my notation I would name it p45f90 -|- p45f90 unit. I have published my way, e.g. in Convention Book 5OSME, Singapour, 2010 , Origami Christmas Book, edited by Anna Kastlunger, 2012 or Convention Book Origami Deutschland, Weimar, 2013.

Phototutorial

StEM can be folded from paper of any aspect ratio, but was designed with square paper in mind. Square paper results in an edge module which is quite bulky and has, in my opinion, better-looking proportions than SEU folded from 2:1 paper which looks a little skinny. Square paper is also closer in line with the classical origami rules.

Finished module and sample usage

Finished module, variants for outwards and inwards facing triangular faces, respectively:

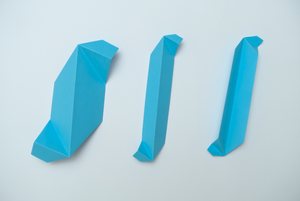
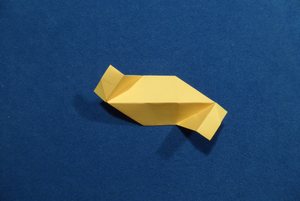
Examples of finished models, using modules made from paper of different proportions:

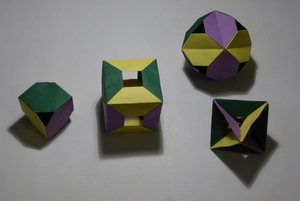
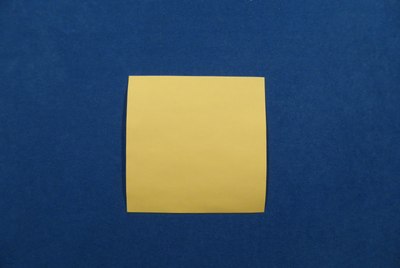
Folding the Sturdy Edge Module

Instructions show folding from a square, but paper of other proportions can be used as well.

1. Start with a square piece of paper.

2. Pleat the square, dividing one side into four equal pieces: first valley fold in half and then mountain fold into halves again.

3. Collapse along the creases. Place the model with two free edges pointing North.

4. Make a crease to divide the width in half. The crease need only go to about 1/3 of the module’s length.

5. Unfold along the main axis and rotate the unit.

6. Valley fold so that the edge of paper lies along the crease created in previous step.

7. Replace the valley fold with an inside reverse fold.

8. Unfold the little corner.

9. Repeat steps 4-8 at the other end of the module. In practice, it may be more convenient to perform each of these steps at both ends one right after another.

10. Crease flaps.

11. Fold along the main axis. For triangular faces, the module’s sides may be made to point inwards or outwards and this will require this one and following folds to be applied in the opposite direction than shown. Images show folding for triangles pointing outwards.

12. Crease flaps for more convenient connection of modules later on.

13a. Unfold. Finished module for outwards-pointing triangles is shown here.

13b. Finished module for inwards-pointing triangles. Many other minor variations are possible and may be required for specific models.

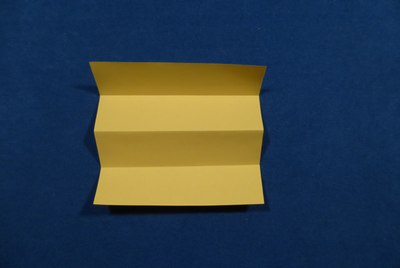
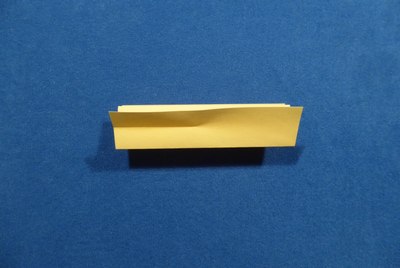
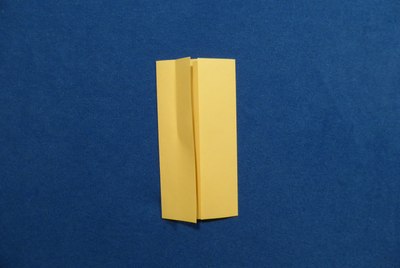
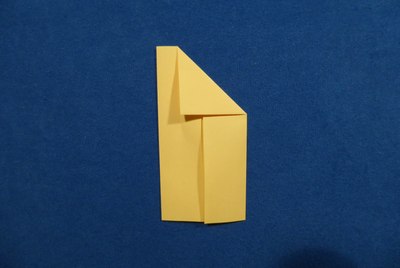
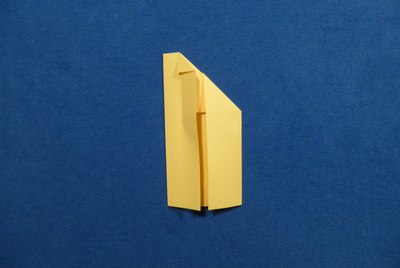
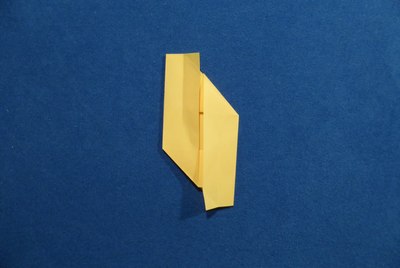
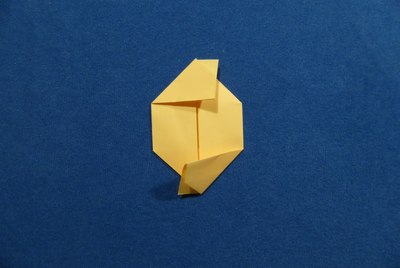
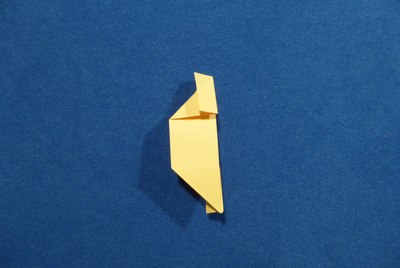
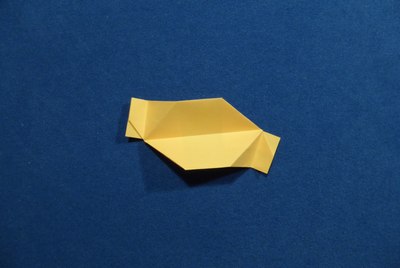
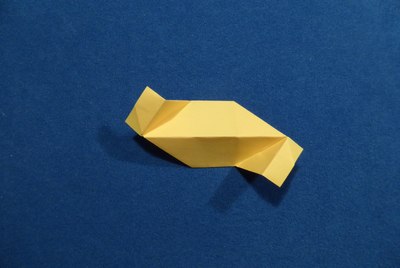
Assembling the modules

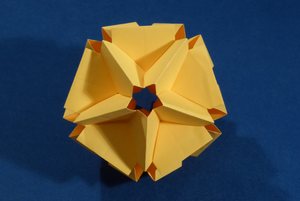
Similar to many other edge units, StEM modules can be connected in many different ways. The angle between the axis and the tip is 45°, so a flat connection results in square faces. If squares are creased along their diagonals you can create spiky balls (as with Sonobe units). Eqilateral triangles can be made with the edge units pointing inwards or outwards, and triangles can be used to create pyramids which cover pentagonal faces. Hexagonal faces can be assembled from six coplanar triangular “faces”.

Additional folds allow the module to be connected into thin and long “belts”. You can find many examples of assemblies using this module at the top of this page.

14. Connecting the modules using flaps.

15a. Flaps fully inserted.

15b. Viewing a larger model against the light reveals how the flaps fit into other units.

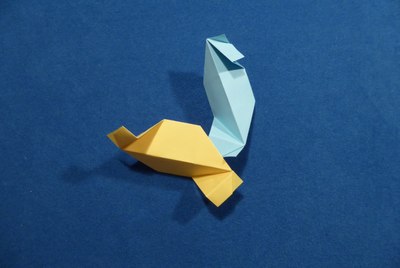
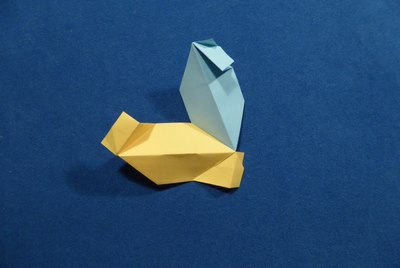
Normally, as in the assembly examples above, StEM is used as an edge unit. However, it can also be folded in half along the shorter axis and used as a face unit. In this setup, it is similar to a Sonobe unit whose sides were cut off. Dave Mitchell invented this “face configuration” (under the name Cube-Corner Star) as early as 1993. An example of this connection method can be seen in the models below.

16a. Connection of StEM “face variant” with Toshie’s jewel model used as an example

16b. Connection of StEM “face variant” with Icosahedron with Inverted Spikes model used as an example

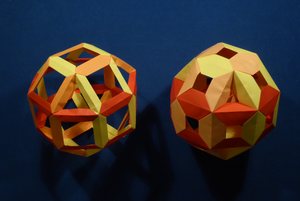
Comparison between SEU and StEM units

In each of these pictures, the model on the left was folded from SEU units, a related design, made of 2:1 paper and the model on the right was folded from StEM units made of 1:1 paper.