Simple Edge Unit (SEU)

SEU, the Simple Edge Unit is an edge unit with a 90° angle at the tip. It can be folded from paper of any proportions and it is quite versatile since with minor modification, it can be used for solids which have almost arbitrary polygons as their faces. After inventing it, I found out that a number of other people had had the same idea before. See the model’s page for details.

Instructions for other peoples’ versions (in print media)

I got the information below courtesy of Dirk Eisner:

Tomoko Fuse’s […]. She has another folding, which is equal to your SEU, in Unit Origami Wonderland. This book is in Japanese. She named it something like 45°-60°-unit. Here the second end of the module is different. Francis Ow named it 90-degrees unit. He has a diagram in his booklet Modular Origami. He started with a 1/6 segmentation, but then it is similar to your unit. With my notation I would name it p45f90 -|- p45f90 unit. I have published my way, e.g. in Convention Book 5OSME, Singapour, 2010 , Origami Christmas Book, edited by Anna Kastlunger, 2012 or Convention Book Origami Deutschland, Weimar, 2013.

Phototutorial

This unit can be folded from paper of any aspect ratio. 2:1 paper is convenient due to the ease of preparing such sheets from square paper, but it results in a somewhat skinny module. Paper with A4 proportions (1 to square root of 2), common in Europe, results in a module with proportions which I find close to perfect.

The angle between this unit’s main axis and the edge of the flap is 45 degrees, so you can fold flat faces of square shape. As with many similar edge units, you can also fold triangular faces from this unit, in which case you can make the module’s sides point either inwards or outwards. Choosing either variant requires minor changes in the way the units are folded.

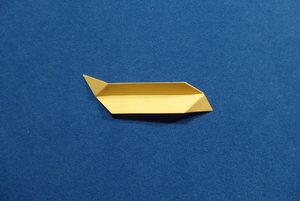
Finished module and sample usage

The finished module pictures are, in order: 2:1 paper (triangles pointing inwards), 2:1 paper (triangles pointing outwards), 1:1 square paper (Sonobe-like variant). Sonobe-like variant can also be folded for assembly with inwards- or outwards-facing pyramids.

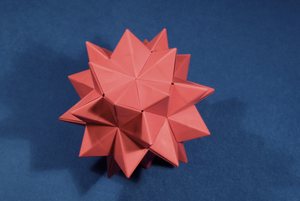
Assembly examples in the picture with multiple polyhedra were folded from 2:1 and 1:1 paper. Notice how the tetrahedron made from Sonobe-like variant of the module becomes a cube. Some bending is visible in the truncated tetrahedron model - this is one of the deficiencies in SEU made from high aspect ratio paper which the Sturdy Edge Module solves.

Other examples are made from different paper proportions, including 2:1, 1:1 square paper (Sonobe-like variant) and 1:√2 (A4 paper proportions).

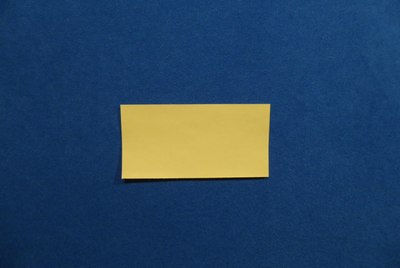
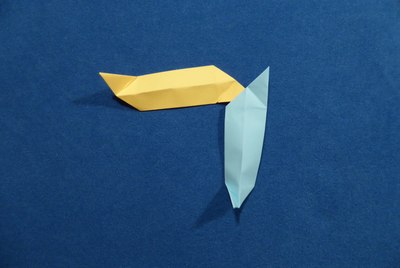
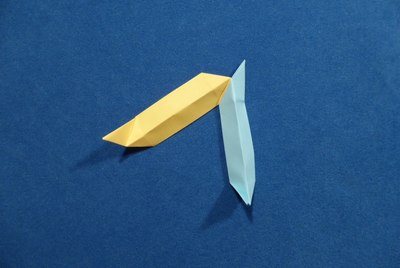
Folding the Simple Edge Unit

Instructions show 2:1 paper but this module can be folded from paper of any proportions. If you start with a square piece of paper, you will get a Sonobe-like variant of the unit.

1. Start with a rectangular or square piece of paper (2:1 shown in the instructions).

2. Pleat, dividing the shorter side into four equal pieces: first valley fold in half and then mountain fold into halves again.

3. Collapse along the creases.

4. Unfold the middle crease, rotate the model as shown in the picture.

5. Valley fold the module’s corner, forming a 45-degree angle (top edge should be aligned with left edge).

6. Replace the valley fold with an inside reverse fold.

7. Tuck the little corner inside.

8. Repeat steps 5-7 at the other end of the module. In practice it may be more convenient to perform each of these steps at both ends one right after another.

9. Crease flaps.

10. Fold along the main axis. For triangular faces, the module’s sides may be made to point inwards or outwards and this will require this one and following folds to be applied in the opposite direction than shown. Images show folding for triangles pointing outwards.

11a. Unfold. This is the finished module for outwards pointing triangles.

11b. Finished module for inwards pointing triangles.

11c. Finished module, folded from square paper (Sonobe-like variant). This is for inwards-pointing triangles; outwards-pointing is also possible.

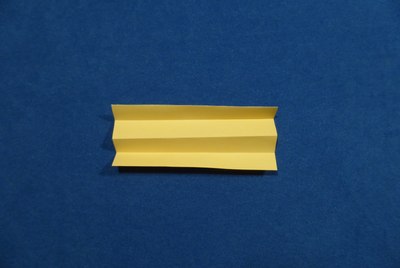
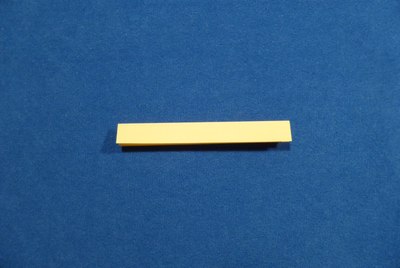
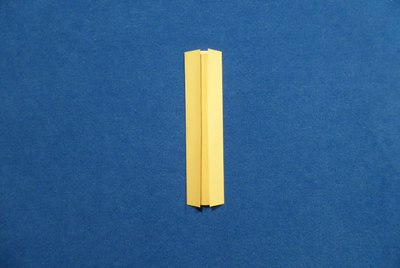
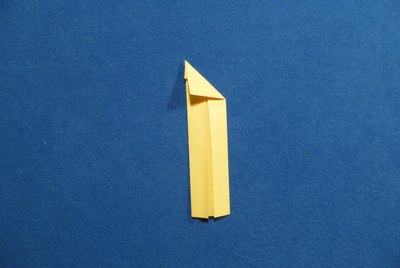
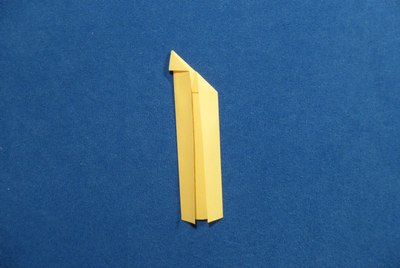
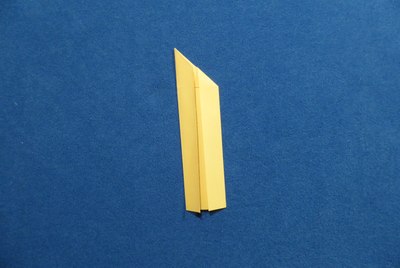
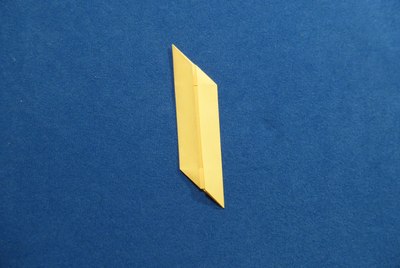
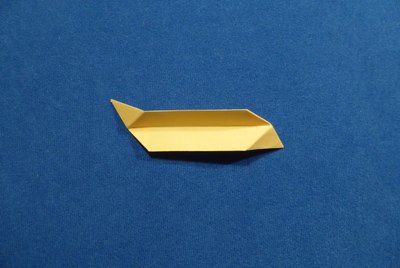
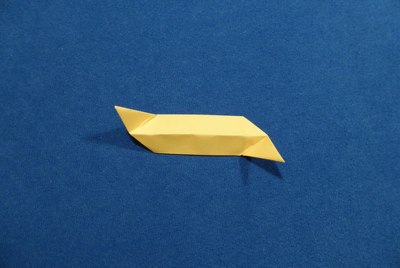
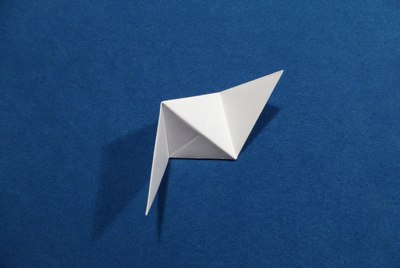
Depending on how modules will be connected, it may be a good idea to add creases on the flaps to make the modules align better against each other. Many other minor variations are possible and may be required for specific models.

Assembling the modules

Similar to many other edge units, SEU units can be connected in many different ways. The angle between the axis and the tip is 45°, so a flat connection results in square faces. If squares are creased along their diagonals, you can create spiky balls (like with Sonobe units). Equilateral triangles can be made with the edge units pointing inwards or outwards, and triangles can be used to create pyramids which cover pentagonal or hexagonal faces. Many other connection methods are possible, such as long, thin belts, and for the Sonobe-like version anything that is possible with Sonobe modules, is also possible with SEU. You can find some examples of assemblies using this module at the top of this page.

12a. Connecting the modules using flaps (2:1 paper).

13. Flaps fully inserted (2:1 paper).

For square paper, there are several different ways of connecting the Sonobe-like units, resulting in different patterns visible in the finished model. See the section on the relation to Sonobe units below for details.

12b. Square paper, “SEU link”

12c. Square paper, “reversed SEU link”

12d. Square paper, “Sonobe link”

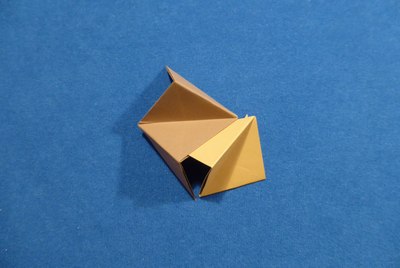
Connection methods and relation to Sonobe units

SEU link

Reversed SEU link

Sonobe link

Comparison of the three connection methods side by side

Rotated link

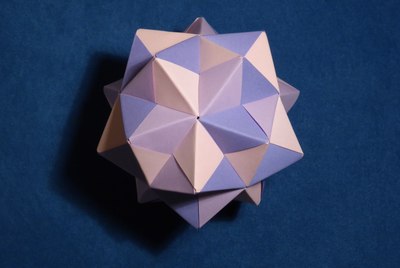
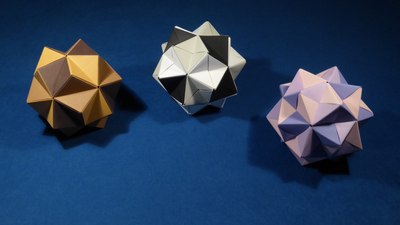
When folded from 1:1 paper, this unit becomes a variant of Sonobe. A peculiarity of this variant is that the module’s pockets extend all the way to the module’s edges. This results in a very stable connection which makes it easy to fold models with high-tension angles. Additionally, one can use either the deep pockets or use the crease on the back face of the module as a pocket and connect the units in several different ways. This is a very nice property since one can create assemblies which look quite differently without modifying the units or their relative composition at all, only by using different connection methods.

In the images here, you can see four different ways of connecting the Sonobe-like variant of SEU unit. Close-ups of individual unit links can be found below, at the end of “folding” section.

These connection methods are:

• SEU link — flaps are inserted into the large pockets, as they would be in units made from elongated paper sheets.
• Reversed SEU link — modules are connected like in regular SEU link, but the reverse faces of modules are on the outside of the model, which results in small creases being visible in the finished model.
• Sonobe link — modules’ reverse faces are on the outside of the model and flaps are inserted into the crease, resulting in a pattern similar to those found in other Sonobe variants.
• Rotated link — instead of valley folding each square’s diagonal, thus each module making up one face for each of two adjacent triangular pyramids, the module’s diagonal is mountain-folded and each module builds one edge of a single triangular pyramid. Thus, the number of units needed for a model is twice as high as for the previously mentioned connection methods. Each module only participates in one pyramid, so each paper patch on the surface of the model can be a different color (as in the example picture) while with the other linking methods, two adjacent patches always share the same color since they are formed from parts of a single module.

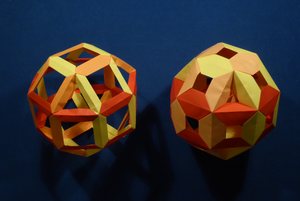
Comparison between SEU and StEM units

From the Simple Edge Unit, I derived another module, the Sturdy Edge Module (StEM) in order to deal with some deficiencies of the original design. StEM is a bit more complex to fold, but has a stronger link and different width-to-length proportions when folded from square paper.

In each of these pictures, the model on the left was folded from SEU units made of 2:1 paper and the model on the right was folded from StEM units made of 1:1 paper.