Sturdy Edge Module (StEM)

Model
…/420842561414931
Folding instructions: Sturdy Edge Module
This is the primary page for this model.
Type: single unit (implies: single-sheet)
Author: Dirk Eisner, Francis Ow, Michał Kosmulski, other/uknown, Tomoko Fuse (independent discovery)
Models using this unit: Cube (StEM), Octahedron (StEM, modules pointing inside), Octahedron (StEM, modules pointing outside), Rhombicuboctahedron, Ring, Tetrahedron (StEM), Truncated Tetrahedron, Icosahedron with Inverted Spikes, Menger Sponge (level 1½), Octahedron with Inverted Spikes, Spiked Icosahedron (StEM face variant), Spiked Icosahedron, Toshie’s Jewel, Truncated Cuboctahedron, 14-Spoked Wheel, Icosahedron with Inverted Spikes, Annapurna, Flower Icosahedron (StEM), Spiked Icosahedron (StEM face variant) from Kami
In albums: Models designed by me and by others, Simple models

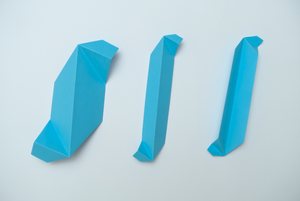
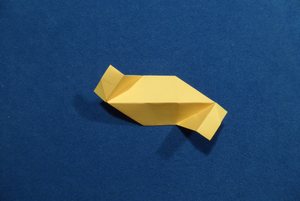
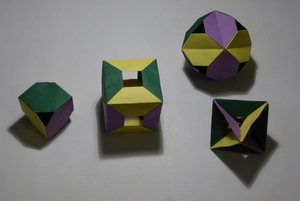
Several unit variants, using different paper proportions Single unit (for triangles facing outwards) Single unit (for triangles facing inwards) Usage example: Tetrahedron, Cube, two variants of Octahedron Usage example: Rhombicuboctahedron Usage example: Truncated Cuboctahedron Usage example: Spoked Wheel Usage example: Annapurna (using StEM instead of Robert Lang’s unit originally designed for this model) Usage example: Icosahedron with Inverted Spikes on All Faces Usage example: Spiked Icosahedron Usage example: Spiked Icosahedron (“face variant” of StEM) 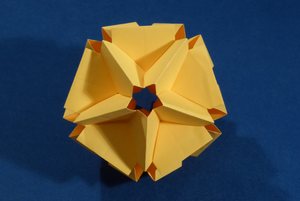Usage example: Icosahedron with Inverted Spikes on All Faces (“face variant” of StEM) Usage example: Menger Sponge Usage example: Ring Usage example: Toshie’s Jewel
Images are licensed under the Creative Commons Attribution-NonCommercial 4.0 International License

Sturdy Edge Module (StEM) was designed by me, and as I later learned, also independently by many other people before. It is a versatile edge unit and a special case of a 90°-generic edge unit. It can be folded from paper with any proportions.

This unit is derived from Simple Edge Unit (SEU), so many of SEU’s properties also apply to StEM. Compared to SEU, it is a bit more complex to fold and the modules are harder to connect, but it offers a number of advantages in exchange. The link between units is stronger and can usually be folded with a neater finish, which is especially visible when faces of a polyhedron meet at very acute or very obtuse angles. StEM can be folded from paper of any aspect ratio, but was designed with square paper in mind. Square paper results in an edge module which is quite bulky and has, in my opinion, better-looking proportions than SEU folded from 2:1 paper which looks a little skinny. Square paper is also closer in line with the classical origami rules.

I designed this unit in November 2014 and published it in December 2014. This unit is quite simple and after designing it, I learned that it had been independently discovered by others before. In particular, Dirk Eisner came up with the same design, and it seems others have as well.

In January 2015 when looking for potential prior art for this unit, I stumbled upon Carmen Sprung’s modular icosahedron design. Her unit was published in 2002 and is very similar to StEM: it looks like a StEM unit folded from square paper with some additional creases. The folding sequence is different from mine even though it leads to a similar final form. Additionally, her version uses only square paper and can only be used to make triangular faces. It seems that the additional creases prevented her from seeing the full potential of the “partially folded” form which would be StEM.

Published: , Updated:

Comments