Steinhaus Puzzle

Model September 8, 2004 April 18, 2021
…/zEkUZY …/z15KoV
Folding instructions: Penultimate Unit
This is the primary page for this model.
Paper: copy paper (note block)
Type: action origami, composition, figurative modular, other modular polyhedron (implies: abstract modular, abstract, balls and polyhedra, figurative, geometric, mathematical object, modular balls and polyhedra, modular, multi-sheet, other polyhedra)
Author: Michał Kosmulski
Units used: Penultimate Unit, by: Robert Neale
Unit count: 240
Colors: multi-colored
In albums: Modular ③ – many units

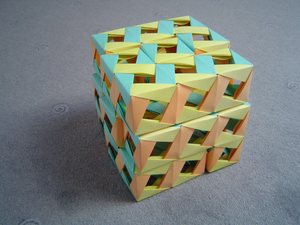
Assembled into a cube — Images are licensed under the Creative Commons Attribution-NonCommercial 4.0 International License

One of many building-like structures one can assemble from the pieces — Images are licensed under the Creative Commons Attribution-NonCommercial 4.0 International License

This puzzle, described in Hugo Steinhaus’ book Kalejdoskop matematyczny (Mathematical Snapshots, literally Mathematical Kaleidoscope) consists of six pieces, which can be assembled together to form a cube (as in the first image) or a variety of different shapes (such as the one shown in the second image). All separate pieces are shown in the last image. A similar puzzle, better known in the English-speaking world, is the Soma cube.

The number of modules in the complete model is 240, with 3 puzzle pieces using 44 modules each and 3 using 36 modules each.

An interesting discussion was started by Ardonik in this flickr thread:

This one’s new. I’m surprised I haven’t heard of it before. Is there any pattern behind the Steinhaus Cube’s individual components?

And here’s what I found out:

I checked Steinhaus’ book and there is no rationale given for choosing this particular set of pieces. Since there are probably quite many different possible ways of devising puzzles of this type, different authors seem to have come up with different divisions of the cube into pieces.

Comments