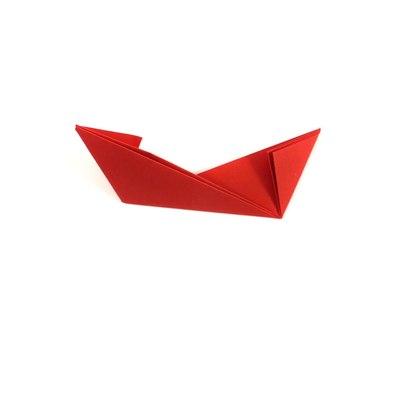
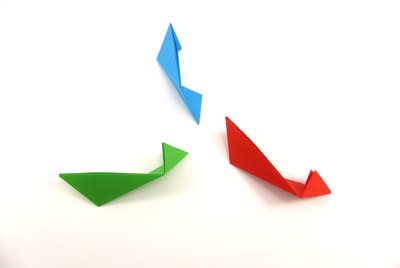
Penultimate Unit: 108° angle for pentagonal faces

The instructions presented here are for the simplest version of the unit, which results in 108° angles and thus can build faces that are regular pentagons. The construction is approximate, but it is good enough for any practical uses. The simplest example of using such units is shown below — building a regular dodecahedron. It has 30 edges and thus requires 30 units. Here are some examples of what else you can build by combining 108° units with other variants (including units with different angles at each end):

• Pentagonal Snowflake
• Truncated Icosahedron
• Dodecahedron with Prisms on All Faces
• Modified Buckyball (uses Penultimate and PHiZZ units)

See other sections of this tutorial for instructions on folding and connecting other variants of this unit.

1. Start with a square

2. Valley fold

3. Valley fold

4. Fold as in previous step on the back

5. Fold down corner

6. Fold the other corner

7. Valley fold the diagonal of the 2:1 rectangle in central part of the module (only one of the two diagonals works!)

8. Module seen from the other side

9. For making a dodecahedron, you will need 30 units

10. This and a few following steps show how to connect three units that form a single vertex

11. Put the tab at the end of a unit inside the pocket formed between layers on the side of another unit

12.

13.

14. A single vertex is complete

15. Same vertex seen from the other side. Now keep adding more units as shown in following images. All vertices have the same structure as this first one.

16.

17.

18.

19. The last vertex may be harder to close than those before, but its structure is the same. Each unit holds its neighbor’s tab and has its own tab inserted into the other neighbor.

20.

21.

22.

23. Last vertex fully closed

24. Complete dodecahedron